The Harmonic Wheel is a new representation of Sounds, where the Consonance Relationships among them are clearly shown. It is, therefore, a powerful tool for the comprehension of Music Theory and Harmony.

The Harmonic Wheel has been developed by considering 2 kinds of Consonance Relationships: Consonant Intervals (2 note consonances) and Consonant Chords (3 note consonances). As it is based on the most fundamental relationships among sounds, its application field covers the different musical styles: classical music, modern music, jazz, latin music, etc. Moreover, it is designed in such a way that, in order to use it, it is not necessary to be able to read music. In order to facilitate its use, diverse examples are given.

As a matter of fact, the Harmonic Wheel is the result of a long research work, which began with a comparative study of the different musical styles in Western Music. In first place, those elements which are common in these styles are taken out; and, after a very long theoretical development, the new representation of sounds is achieved. As a research work, it is being published in some specialized and prestigious journals (see the section PUBLICATIONS).

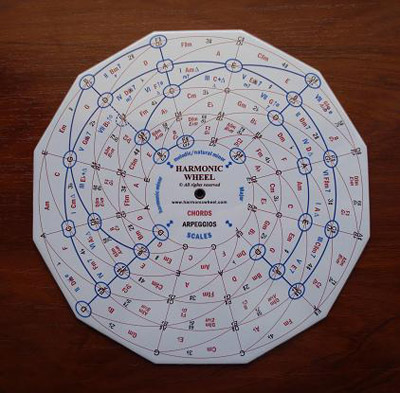
The HARMONIC WHEEL has many practical applications, most of which are described in the next chapters. A non-exhaustive listing of the principal questions that can be solved with this powerful and versatile abacus is included below.

1. Enharmonic notes and chords.
2. Given a note, obtaining the 6 notes with which it forms consonant intervals.
3. Obtaining the interval between any two notes.
4. Notes of every Major Scale.
5. Notes of every minor Scale (harmonic, melodic or natural).
6. Key Signature of any Major or minor Scale.
7. Map of the Keys: Cycle of Fifths and Changes of Mode.
8. Arpeggios of any Major or minor chord.
9. Given a note, obtaining the 6 consonant chords (Major and minor) containing it.
10. Arpeggios of any Augmented or diminished chord.
11. Simple graphical representation of all possible 3 and 4 note chords built by superimposing Major and minor thirds. Finding their arpeggios.
12. Chord Finder: Chords associated to any Major or minor scale (harmonic, melodic or natural).
13. Graphic visualization of the chords which are more affine or less affine to a given scale.
14. Transposition of a chord progression.
15. Simple graphical representation of Pentatonic Scales and deriving their main properties.
16. Simple graphical representation of diminished and Hexatonic Scales. Finding their associated chords.

In the APPLICATIONS OF THE HARMONIC WHEEL section some more advanced aspects are included, like several modulation techniques, the Béla Bartók's axis system or the Coltrane changes in Jazz.

VIDEOS HARMONIC WHEEL:

BUY NOW THE HARMONIC WHEEL